Bas d'Herripon and Jan Verhoeven's drivetrain loss measurements
In Human Power 48 (1999), Dave Wilson does a nice review of posts to the now defunct Bicycling Science mailing list (previously called "Hardcore Bicycle Science", presumably named to discourage the usual idle chatter which had diluted the rec.bicycles.tech USENET group). The most relevant data to my purpose was credited to Jan Verhoeven, although details were not available.
Searching the list archives, I found similar data from Bas d'Herripon.
Both tests were on a Shimano Deore LX drivetrain. Here's the results from d'Herripon:
For d'Herripon, crank cadence was at 70 rpm. I assume the same for Verhoeven. Both data sets were for drive power of 200 watts.
My model has three unknowns: K, T0, and Kd. But as before, I can set Kd based on specific measurements, using a value of 94 mJ/revolution measured on Dura-Ace pulleys. Then there's two unknowns for me to tweak to match the data.
Well, I did that using an OpenOffice Calc spreadsheet. But it became quickly clear to me I was in trouble. Look at the range of those efficiencies: from 91.6% to 98.2%. This is a range of drivetrain loss coefficients from 1.8% to 8.4%, a range of 467%! Nothing in my model allows for such a strong dependence on gear selection, given the range of chainrings and cogs used in this test.
In desparation, I plotted drivetrain losses versus gear ratio, and was surprised to see the following:
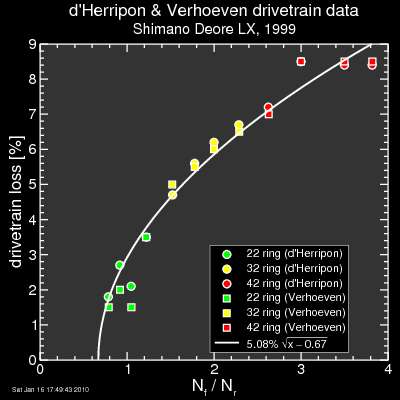
Drivetrain losses appear to be primarily a function of the gear ratio, rather than of some other function of the front and rear chainring, such as my suggested 1/Nf + 1/Nr for tension-dominated losses.
Note in the plot I fit a function to the data. I can't help it -- I like fitting functions to data. But there's no physics behind that function: it's simply what came to my head when I was looking at the data.
Since cadence is fixed, the rear wheel is spinning at a rotation rate equal to that cadence (70 rpm) times the gear ratio, so from this it appears drivetrain losses were a function of the rate the rear wheel was spinning. That's the sort of thing I'd expect from bearing or tire losses or wind resistance from the spokes, not chain losses.
Now, d'Herripon claims they corrected for spoke wind resistance. But I'm going to guess they made an error in doing so. Just a guess.
So first Spicer, then Kyle and Berto, and now Verhoeven and d'Herripon: I can't figure any of them out.
Searching the list archives, I found similar data from Bas d'Herripon.
Both tests were on a Shimano Deore LX drivetrain. Here's the results from d'Herripon:
For d'Herripon, crank cadence was at 70 rpm. I assume the same for Verhoeven. Both data sets were for drive power of 200 watts.
My model has three unknowns: K, T0, and Kd. But as before, I can set Kd based on specific measurements, using a value of 94 mJ/revolution measured on Dura-Ace pulleys. Then there's two unknowns for me to tweak to match the data.
Well, I did that using an OpenOffice Calc spreadsheet. But it became quickly clear to me I was in trouble. Look at the range of those efficiencies: from 91.6% to 98.2%. This is a range of drivetrain loss coefficients from 1.8% to 8.4%, a range of 467%! Nothing in my model allows for such a strong dependence on gear selection, given the range of chainrings and cogs used in this test.
In desparation, I plotted drivetrain losses versus gear ratio, and was surprised to see the following:

Drivetrain losses appear to be primarily a function of the gear ratio, rather than of some other function of the front and rear chainring, such as my suggested 1/Nf + 1/Nr for tension-dominated losses.
Note in the plot I fit a function to the data. I can't help it -- I like fitting functions to data. But there's no physics behind that function: it's simply what came to my head when I was looking at the data.
Since cadence is fixed, the rear wheel is spinning at a rotation rate equal to that cadence (70 rpm) times the gear ratio, so from this it appears drivetrain losses were a function of the rate the rear wheel was spinning. That's the sort of thing I'd expect from bearing or tire losses or wind resistance from the spokes, not chain losses.
Now, d'Herripon claims they corrected for spoke wind resistance. But I'm going to guess they made an error in doing so. Just a guess.
So first Spicer, then Kyle and Berto, and now Verhoeven and d'Herripon: I can't figure any of them out.

Comments