On Bicycles, and.... what else is there?

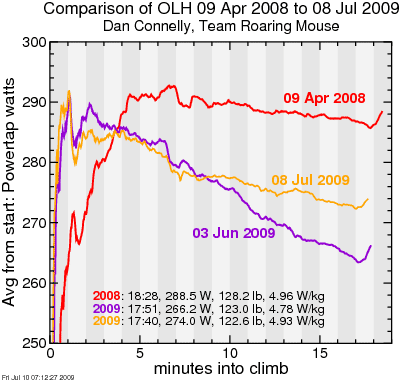
VAM is an easy to calculate proxy for power-to-mass ratio commonly reported for professional riders in European races, where television coverage plus well documented hill profiles facilitates its extraction. VAM is the rate of altitude gained per unit time, for example in meters per hour. I recently recorded 1402 metters per hour in a break-through ride up Old La Honda, breaking my previous PR. The excellent Science of Sport Blog recently posted the following plot of some VAMs from recent Tour history: VAMs from the Tour de France, from The Science of Sport . Michele Ferrari's numerical calculation of the effect of road grade on VAM are often used as a reference, by me included, but an analytic form is perhaps more useful. I'll again assert a simplified, windless bike power-speed model, which is for "PowerTap power" neglecting drivetrain losses, but it is assumed drivetrain losses are a function of total power: p = f m m s + f w s³ , where p is a fixed power,

Well, racing this year has gone terribly. There's really no other way to put it. In road races, DNF's in Spring Hill and Pescadero. Crashed out of Berkeley Hills. Dropped at Mt Hamilton and Diamond Valley. This isn't what we train for. It'd have been better to focus on double centuries. I've done better on hillclimbs: Diablo as solid a 5th out of 6 as you can get, and a satisfying 2nd Ross' Epic. Small fields in each case, but the guys who beat me are guys who should be beating me. I rode well in each case. But besides racing, my goal this year (as last year) was to crack 17 minutes up Old La Honda. My existing PR has been 17:03 from 2002. That was on a sub-16 lb Fuji Team, actually a Teschner hand-built bike for the Mercury team. The frame cracked on me last year, and I replaced it with another Fuji, this time a real Fuji, the SL/1 carbon frame, which I love. Weenied out it weighs in at a very nice 11.35 lb. So with that sort of advantage, I certain

I came, I saw, it kicked my ... The Diamond Valley Road Race hit me on Saturday like a punch in the gut. Our well-tempered pack of not much more than 30 riders started peacefully enough: we rode civilly from starting area to begin the first of five laps of the 11.4 mile course. My goal: stay near the front to have a good position on the subsequent climb. This is something I'd failed to do each of my previous two rides here, and in each case it cost me added anaerobic stress during the beginning of the two-part climb. In each case I'd managed to survive these efforts, move up during the race, and exploit general attrition to place (for me) fairly well. But it's always easier climbing from the front than the back: position on a climb this short is money in the bank worth more than any weight-weenie gram shaving. I'd arrived with Cara to our campground late Friday afternoon. A solid night's rest camping at Grover Hot Springs, then a relaxed breakfast, and I still

I calculated the effect of body mass on wind resistance , and previously the effect of wind resistance on climbing speed , and before that the effect of total mass on climbing speed , so these three analyses can be combined to calculate the total effect of body mass change on climbing speed. Assuming body mass changes are relatively small, the effect of a body mass change on wind resistance can be linearized. Assuming the wind resistance associated with the body, initially a fraction of total wind resistance β w . Then a fractional change ε w of the body's wind resistance will have an effect on total wind resistance = ε w β w . Assuming the change in the body's wind resistance is due to mass change at a fixed height, this fractional wind resistance change ε w is to first order half the fractional change in body mass ε m (as a result of the square root). So, if δ w is the fractional change in total wind resistance, it can be calculated: δ w = ε m β w / 2 . If f is th

I already considered the effect of mass on climbing speed , but body mass (as opposed to equipment mass) also affects wind resistance, which is still important when climbing . This has been estimated empirically by Heil, European Journal of Applied Physiology , V 93 N 5-6; March, 2005 . However, I never really liked Heil's analysis, because it looks at body mass without also considering body height. Obviously if you consider gaining mass without height versus gaining mass in proportion to height it is reasonable to expect different results. I'm reminded of a physics lecture I attended as an undergrad: "First, we model the human body as a sphere." The crowd chuckled. It was a sphere of salt water, BTW, useful for electrostatic calculations, but of course the human body is not a sphere. So shape matters. So first the usual wind resistance model, in the absence of external wind: P w = f w s³ f w = ½ ρ C D A , where ρ is the mass density of air, C D is the wind

To continue the previous analysis: what about the effect of wind resistance on climbing? Well, the result should be easily derived from the last two analyses. But for completeness, starting again from the simple power-speed model, assuming zero wind: p = f m m s + f w s³ we can then calculate the derivative of the dependence of wind resistance on subsequent speed s, where we're interested in fractional rather than absolute changes of each: (f w / s) d s / d f w = (f w / s) (∂ p / ∂ f w ) / (∂ p / ∂ s) where: ∂ p / ∂ f w = s³ , ∂ p / ∂ s = f m m + 3 f w s² . So: (f w / s) ∂ s / ∂ f w = (f w / s) s³ / ( f m m + 3 f w s² ) = f w s² / ( f m m + 3 f w s² ) which can be expressed as: r / ( 1 + 3 r ) where r is the ratio of wind resistance power to mass-proportional power. If you know instead the fraction of total power (neglecting drivetrain losses) due to wind resistance, then substitute r = f / (1 - f), where f is this fraction: f / ( 1 + 2 f ) . So back to our previo

Similar to the last post, the next question is: what is the effect of power on climbing speed? Answering this is a fairly trivial extension of the previous calculation. Again consider a power-speed model which, simplified and neglecting wind speed and acceleration, is expressed as: p = f m m s + f w s³ where terms are as described last time. Then we can calculate the effect of fractional changes in speed on fractional changes in power required: (s / p) (∂ p / ∂ s) = (s / p) ( f m m + 3 f w s² ) . What we want, however, is the inverse of this: the effect of fractional changes in power on fractional changes in speed: (p / s) / ( f m m + 3 f w s² ) . Expanding p / s yields: ( f m m + f w s² ) / ( f m m + 3 f w s² ) which can be simplified in terms of the ratio of wind resistance to mass-proportional power used in the last blog entry: (1 + r) / (1 + 3r) . Again, if what we know isn't the ratio of wind resistance power to mass-proportional power, but rather the ratio of wi

A frequent question in cycling speed-power calculations is: "If I save a certain percent of weight, then what is the effect on my speed?". The same ananalysis applies to other mass-proportional power terms: the effect of grade and/or rolling resistance changes on speed. This has tangible significance when considering how much it's worth to invest in some über-light weight-weenie widget, or when considering the trade-off between aerodynamic versus mass in wheels or frames. Consider a power-speed model which, simplified and neglecting wind speed and acceleration, is expressed as: p = f m m s + f w s³ where f m is the mass-proportional coefficient and f w is the wind resistance coefficient. In the standard linearized model, f m = g (grade + C rr ) / α, where g is the acceleration of gravity, grade is the road grade, C rr is the coefficient of rolling resistance, and α is the drivetrain efficiency (characterizing the drivetrain as a power-proportional loss term may b

Note: I had to revise the numbers on this page because mapmyride does a relatively poor job with distance, as I determined by considering its profile of Old La Honda Road. Since I did the original calculation, an official km-by-km climb profile was posted to The Science of Sport . The Tour today ended with a relatively steep, short climb on a stage on which riders had relatively fresh legs: a perfect opportunity for some truly impressive rates of climbing to be demonstrated. And sure enough, they didn't disappoint. Various numbers have been tossed about for the stats on Verbier. Originally I used elevation data from MapMyRide's map of the stage : 630 meters climbed over 7.576 kilometers starting from the sharp left from Route de Verbier at km 199.1 in the stage, an average gradient of 8.32%. However, since then official data were posted in comments to The Science of Sport : 638 meters gained over 8.8 km, an average grade of 7.25%. They also reported reports that Contador d

A recovery week for me this week, a time to reflect on my fitness, my training, and my priorities. A chance to avoid digging myself into a metabolic hole, something I've done too many times before, and something which so many cyclists invariably do in the endless season which is San Francisco Bay area cycling. Hammering is an addiction, as strong as any, as potentially self-destructive as most. This time for recovery was deflected from its canonical trajectory by the news of Chris Hipp's sudden death . It even cracked the New York Times , and not because of his cycling prowess. Yet despite his fame, of which I was unaware, Chris who was always a mystery to me. My most recent impression of Chris is from the Noon Ride, when I was intrigued by his Leopard frame . Cyclists can generally be binned into two groups: those who obsess about equipment and those who don't. I'm definitely among the former. When I see an interesting frame, I want to know everything. How does

So, the word is that Fremont Peak will get the Mt Tam spot on the calendar this year, as Mt Tam had resistance due to the threat of park closure due to the California State "budget crisis". Okay, political commentary. Skip to next paragraph. I wouldn't be surprised if shutting the state parks for a few weeks actually cost more money than it saved. The parks are already fairly poorly funded. It's obviously political posturing to dangle some potential for pain in front of the People so that when the pain is withheld, the "mild discomfort" which remains is better tolerated. Okay, end of political commentary. View from Fremont Peak ( Virtual Tourist ) But with the calendar spot taken by a Fremont Peak climb, although those of us in San Francisco and northern environs are inconvenienced by the additional distance, on a climb-for-climb basis I'd have to say Fremont Peak wins. I've never been up Fremont Peak, but thanks to the incredible data resour

I love it when the break stays away. And what a classic sprint! A regression of the trend to 5 km to go was a 3 second gap at the finish, but then Caisse d'Epargne basically gave up the chase.

I exited the BART in Walnut Creek to find Anthony getting off the same train. I always have difficulty finding my bearings outside the Walnut Creek station, so welcomed having someone to follow. We were both there for the same purpose: the House of Pain . Well, almost.... he was doing "House of Pain Lite", a less maniacal version of the ride. Or should that be "The House Of Mild Discomfort"? Maybe another time for me. Today I wanted to throw myself at the mercy of the real deal. Ben's spoken highly of it: an East Bay hammerfest, a chance to acquire some heat adaptation not likely available from Roasters. We rode together to Danville Peet's , arriving around 8:40 am. A decent group was there for the Lite ride, and they were soon gone. I waited basically alone for a group to congeal for the main ride, analogous to the group which picks up Spectrum from Los Altos Peet's , but nothing really formed. I guess most of the riders go to the Park and Ride, or mee

Tuesday : 20-50 second sprints on Potrero Hill. I love this sort of workout. Quality pain time. But for much of it I was still chilly in my arm warmers, knee warmers, and vest. A typical summer evening in San Francisco. Wednesday : Finally, progress! 274 PowerTap watts up Old La Honda. Only 0.6% lower W/kg than my 2008 best power up the same hill. Given the leg-thrashing I experienced the previous evening, I think I have a few % more in me. My time of 17:40 was very solid considering I was on my steel Ritchey Breakaway with heavy wheels: there's still hope for my sub-17 minute goal. Indeed, calculations show were I on my weenied SL/1, I would have done it today. But calculations don't count. Really, I'd like to break 17 by a sufficient margin for calculations shown I would also have done so on my 16 lb Al Fuji Team I rode in 2002. Given running this past offseason left me at a lower base-line body mass than I've had for years, I just need to keep improving

Fellow Mouse PPH was driving us to Leesville on Friday. Despite our 5:05 am departure, it was getting warm in the car as we passed through Vacaville on I-80. I checked in the back for my bag, in which I'd stored my water bottles for the day, jammed in there admist my ride clothing, shoes, and food. No bag. Whoops. I'd left it at his place when I'd gone in to use his toilet. I'd be doing support duties for this race... Which turned out to be a lot of fun, and useful on a course where flats are common and the feed zone is essential. If nothing else, being in the feed zone meant I could hand-pick which of the reused discarded bottles the neutral feed was handing out I could give to my teammates. Maybe save them from swine flu. No complaints about the neutral feed, BTW: nobody forces any rider to take neutral water. Beggers can't be choosers. So while the day was productive, it didn't do anything for my fitness, obviously. Unless sweating in a hot feedzo

I've been doing a lot of intervals lately... 10 second, 15 second, 30 second, 5 minute. As a result, the left side of my maximal power curve has filled in nicely compared to last year. Maximal power curve through 04 June, curve for June alone, and curve for surviving data from 2008 You can really see from the curve that the curve using June data is notably improved from earlier in the year: approaching the 2008 numbers. The exception is sprints, but the sprint session in question was late May, virtually June, so is part of the same trend of improvement. An aside: one thing I've seen with my meter is that if the hill requires a lot of shifting, if the grade varies a lot, average power is going to be lower. It's just harder to sustain a constant effort if the road itself isn't sufficiently constant. The obvious extreme of this is an intermediate descent on a hill. Even a small one is a kiss of death for posting up a good point on the maximal power curve. I've r

I started with the Nooner yesterday, then split off @ Golden Oak to do 30 second sprints. First I did four on various hills around there, then I headed up Alpine, planning on doing more on the climb towards the gate where I'd turn around. A small deviation on Alpine Trail led to some hiking around the numerous railroad ties, but soon I was back on Alpine, riding past Willowbrook. As I gently climbed I was intrigued by the open house sign at the entrance to a private road. Cool: a chance to check out what's hidden behind the tree cover. But the road, which I'd assumed was a short access route to a few oversized domiciles, instead went on and on and on. I climbed slowly, except every 6 minutes or so doing another 30 second sprint. On my third sprint, I finally reached the " open house ". I didn't see much of a house, only a driveway, but instead of investigating that further, I continued down the road. Then I came across the colossal compound which is the